Following my longest lesson ever, my shortest lesson ever! 

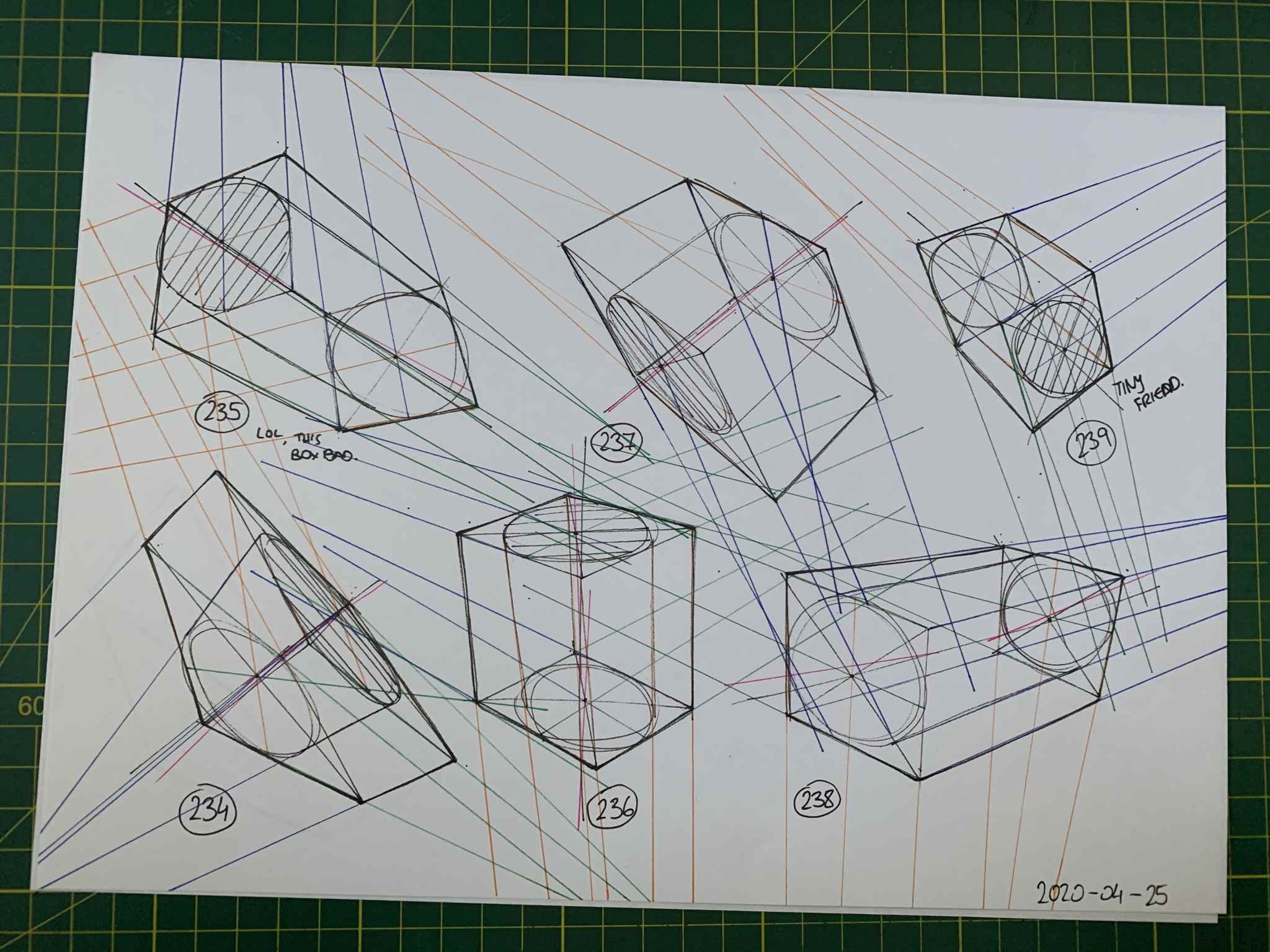
The task: Draw 250 cylinders - 150 of those on an arbitrary minor axis, 100 constructed within a box. This was another one of those lessons where I wasn’t really sure if I was learning till I got to the very end. A bit like the 250 box challenge. It also exposed some serious flaws in my ability to draw boxes.

Surprisingly, both the 150 arbitrary and 100 box cylinders took me roughly the same amount of time - 9 and 10 days respectively. 

Once I got past the arbitrary cylinders, I made a habit of doing at least one page of cylinders in boxes per day because from my experience with the 250 box challenge, I knew drawing that many boxes was going to be a slog. Interestingly, despite becoming quicker at drawing my boxes, my tolerance for the number of boxes I could draw in one session has not increased - I still struggle somewhat to draw more than 10-15. 

The arbitrary cylinders definitely felt a bit haphazard at times and thus I was able to get through dozens of them every session. Judging by the critique I received however, I didn’t do as badly as I felt with these and picked up on the relationship between foreshortening and the minor axis.

250 Cylinder Challenge by the Numbers

You’ll notice I did 4 cylinders in boxes early on, I asked for clarification on whether this was okay to do and got confirmation that the challenge was designed to be done arbitrary first, cylinders in boxes second. That was unfortunate because I did feel like I would’ve felt less burnt out on the cylinders in boxes if I’d been able to interleave them amongst the others. I understand the rationale for it however.

The big goal for the cylinders in boxes wasn’t actually to draw nice cylinders - it was to get practice drawing boxes with square faces. Square faces on a box means that any ellipse that is drawn within them and aligned to the minor axis will be a perfect circle tilted in 3D space. A perfectly drawn box with square faces should then optimally translate to a perfect cylinder. However, any minor imperfection is amplified by the check lines, as seen in the cover image for this post, where a number of the check lines across the contact points for the ellipses skew off in odd directions because the faces of my boxes aren’t perfect.

Overall, I think the challenge was useful, though it’s yet to be seen if I can apply this well to lesson 6 and onwards.

250 Cylinder Challenge